Technologies: Difference between revisions
(no need to keep old info, replaced table with template) |
(moved infinite tech section here from research − the divide between the pages is not really clear, so please argue this decision if you disagree) |
||
| Line 1: | Line 1: | ||
{{Languages}} | {{Languages}} | ||
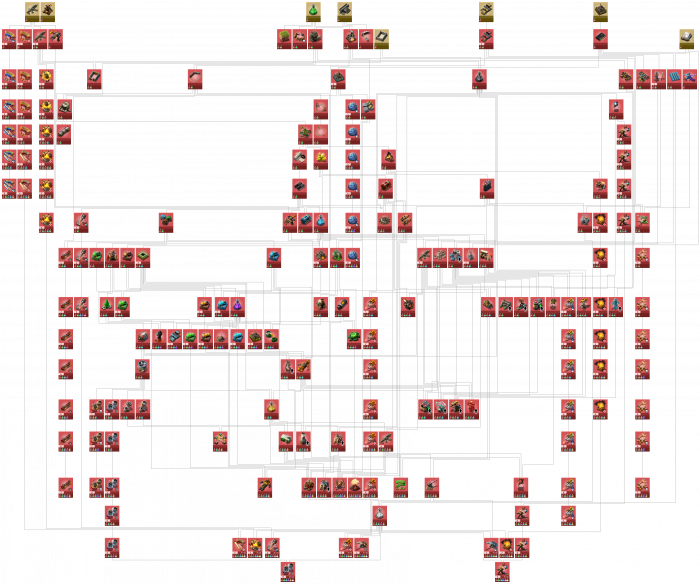
Technologies are [[Research|researched]] in [[lab]]s using [[science pack]]s. | [[File:Technology tree.png|thumb|right|700px|The technology tree − click to enlarge]] | ||
'''Technologies''' are [[Research|researched]] in [[lab]]s using [[science pack]]s. | |||
Every technology needs a certain amount of science packs per science pack type to be researched, for example the [[ | Every technology needs a certain amount of science packs per science pack type to be researched, for example the [[Logistics (research)|Logistics]] technology requires 20 [[science pack 1]] to be placed in labs while the technology is selected for research. | ||
== Achievements == | == Achievements == | ||
{{Achievement|tech-maniac}} | {{Achievement|tech-maniac}} | ||
* Completing infinite technologies of any level is not required for '''Tech maniac'''. All non-infinite levels of technologies that have infinite continuations are still required. | |||
{{clear}} | |||
== Infinite technologies == | |||
While most technologies in ''Factorio'' are either one-off or have a finite, relatively small number of levels available, 14 are "infinite", meaning the player can research as many levels as they can afford. All of them unlock bonuses to existing technologies, never new structures or abilities. The per-level bonuses are constant for a particular infinite technologies and, like finite research bonuses, are additive within a single technology. | |||
All infinite technologies levels require [[space science pack]]s, and are also the only technologies that do. As such, they are late-game technologies intended primarily for players who wish to continue playing and expand their factory past the nominal victory condition of launching a single satellite inside a rocket. | |||
Infinite technologies are identified in-game by a small <code>∞</code> infinity symbol shown in the top right corner of the research technology's card in the research screen. | |||
Most infinite technologies are continuations of ordinary multi-level technologies; the "infinite" mechanic becomes effective once the player reaches the card initially labeled with <code>N - ∞</code> in the research tree. Only the two [[artillery]]-related technologies (artillery shell [[artillery shell range (research)|range]] and [[artillery shell shooting speed (research)|shooting speed]]) are infinite-only; for these, <code>1 - ∞</code> is shown before any levels in them are researched. In either case, once the first infinite level is researched, the card label switches to the one discussed above. | |||
=== Pricing equations === | |||
The price of all infinite technologies is generated in a [[Wikipedia:Progression|mathematical progression]]; for the majority of technologies, the progression is [[Wikipedia:Geometric_progression|geometric]], mostly in powers of 2; a single technology - [[artillery shell shooting speed (research)|artillery shell shooting speed]] - uses a powers-of-3 progression. Two technologies - [[mining productivity (research)|mining productivity]] and [[follower robot count (research)|follower robot count]] - use an [[Wikipedia:Arithmetic_progression|arithmetic progression]] instead, in both cases with a difference of 100. | |||
The equations that generate infinite research prices take the following forms: | |||
#<code>P[N] = P[0] × 2 ^ (N - N[0]) + C</code> - most infinite technologies | |||
#<code>P[N] = P[0] × 3 ^ (N - N[0]) + C</code> - '''artillery shell shooting speed''' | |||
<!-- ** specifically, the coefficients are P = 1,000; N[0] = 0; C = -1,000 --> | |||
#<code>P[N] = P[0] × (N - N[0]) + C</code> - '''mining productivity''' and '''follower robot count''' | |||
where: | |||
*'''''P[N]''''' is the price of the '''''N'''''th level of the technology (as reported by the game; i.e., counting also all starting "non-infinite" levels, if any) | |||
*'''''P[0]''''' is a price multiplier | |||
*'''''N''''' is the level of the technologies, as reported by the game (i.e., including any starting non-infinite levels) | |||
*'''''N[0]''''' is a shift factor relative to the source series | |||
*'''''C''''' is a constant | |||
Among others, the following properties can be observed: | |||
*<code>P[0] = 1,000</code> for technologies with a geometric progression and <code>P[0] = 100</code> for technologies with an arithmetic progression | |||
*for both infinite-only technologies, <code>P[1] = 2 × P[0]</code> | |||
*denote <code>F</code> the final level of non-infinite research available in the technology if such exists; for infinite-only research, set <code>F = 0</code>; then: | |||
*the majority of geometric-progression technologies have <code>P[F+1] = P[0]</code>; the rest have <code>P[F+1] = 2 × P[0]</code> (where ''P[F+1]'' is the price of the first "infinite" level) | |||
*for technologies with preceding non-infinite levels, the relation between ''P[F+1]'' and non-infinite level prices may vary, but in all cases <code>P[F+1] >= P[F]</code> (where ''P[F]'' is the price of the last non-infinite level) | |||
*either <code>N[0] = F</code> or <code>N[0] = F + 1</code>; this is mostly idiosyncratic to the technology in question | |||
*<code>C != 0</code> only for '''follower robot count''' (''C'' = 900) and '''artillery shooting speed''' (''C'' = 1,000) | |||
=== Price table === | |||
The table below summarizes the applicable equation type and parameters (using the same notation as in the preceding section), plus the per-level bonus, for all infinite technologies in the game. Technologies are ordered based on similarity of the parameters; if parameters are identical, then alphabetically. | |||
{| class="wikitable" | |||
! Technology !! Equation <br/> type !! F + 1 !! P[F + 1] !! Equation !! Bonus | |||
|- | |||
| {{Icontech|Gun turret damage (research)}} [[Gun turret damage (research)|Gun turret damage]] || 1 || 7 || 1,000 || 1,000 × 2^(N - F - 1) || +70% | |||
|- | |||
| {{Icontech|Rocket damage (research)}} [[Rocket damage (research)|Rocket damage]] || 1 || 7 || 1,000 || 1,000 × 2^(N - F - 1) || +50% | |||
|- | |||
| {{Icontech|Bullet damage (research)}} [[Bullet damage (research)|Bullet damage]] || 1 || 7 || 1,000 || 1,000 × 2^(N - F - 1) || +40% | |||
|- | |||
| {{Icontech|Shotgun shell damage (research)}} [[Shotgun shell damage (research)|Shotgun shell damage]] || 1 || 7 || 1,000 || 1,000 × 2^(N - F - 1) || +40% | |||
|- | |||
| {{Icontech|Flamethrower damage (research)}} [[Flamethrower damage (research)|Flamethrower damage]] || 1 || 7 || 1,000 || 1,000 × 2^(N - F - 1) || +20% | |||
|- | |||
| {{Icontech|Worker robot speed (research)}} [[Worker robot speed (research)|Worker robot speed ]] || 1 || 6 || 1,000 || 1,000 × 2^(N - F - 1) || +65% | |||
|- | |||
| {{Icontech|Combat robot damage (research)}} [[Combat robot damage (research)|Combat robot damage ]] || 1 || 6 || 1,000 || 1,000 × 2^(N - F - 1) || +30% | |||
|- | |||
| {{Icontech|Cannon shell damage (research)}} [[Cannon shell damage (research)|Cannon shell damage]] || 1 || 6 || 1,000 || 1,000 × 2^(N - F - 1) || +30% | |||
|- | |||
| {{Icontech|Laser turret damage (research)}} [[Laser turret damage (research)|Laser turret damage]] || 1 || 8 || 1,000 || 1,000 × 2^(N - F - 1) || +70% | |||
|- | |||
| {{Icontech|Artillery shell range (research)}} [[Artillery shell range (research)|Artillery shell range]] || 1 || 1 || 2,000 || 1,000 × 2^(N - F) || +30% | |||
|- | |||
| {{Icontech|Grenade damage (research)}} [[Grenade damage (research)|Grenade damage]] || 1 || 7 || 2,000 || 1,000 × 2^(N - F) || +20% | |||
|- | |||
| {{Icontech|Mining productivity (research)}} [[Mining productivity (research)|Mining productivity]] || 3 || 16 || 1,500 || 100 × (N - 1) || +2% | |||
|- | |||
| {{Icontech|Follower robot count (research)}} [[Follower robot count (research)|Follower robot count]] || 3 || 7 || 1,000 || 100 × N + 900 || +10 | |||
|- | |||
| {{Icontech|Artillery shell shooting speed (research)}} [[Artillery shell shooting speed (research)|Artillery shell shooting speed]] || 2 || 1 || 2,000 || 1,000 × 3^(N - F - 1) + 1,000 || +100% | |||
|} | |||
=== Affordability === | |||
Infinite technologies are essentially an inexhaustible resource sink for players who build very large bases. While the bonuses they provide can significantly improve the player's capabilities (particularly as regards combat), they are subject to diminishing returns; thus, the per-level contributions from very high levels of infinite technologies will eventually provide only marginal improvements. | |||
As the price of most infinite technologies (specifically, those based on geometric progressions) increases very steeply, it may be a good idea for players to set realistic target levels for each of the infinite technologies they wish to pursue, and make their factory plans accordingly. To that end, the following properties of cumulative infinite research prices may be useful: | |||
#The cumulative price of the first <code>N - F</code> levels (notation as in previous sections; i.e., here counting "infinite" levels only) of infinite technologies whose underlying equation is a powers-of-two geometric series (see equation type (1) in preceding sections) is <code>2 × P[N] - P[F+1]</code>; i.e., twice the price of the final researched level, less the price of the first "infinite" level. | |||
#*This tends toward <code>2 × P[N]</code> as ''N'' goes to infinity. | |||
#*The above also shows that, assuming constant research speed (usually, this is the same as science pack production capacity), each subsequent level of an infinite technology of this type will take about as long as ''all'' preceding infinite levels took combined (or, twice as long as the previous level). | |||
#*Further, assuming one has reached a level <code>M</code> they consider the "highest feasible" with their current science pack production capacity, expanding said capacity by a factor of '''''X''''' will allow at least <code>floor(log[2](X))</code> and at most <code>ceiling(log[2](X))</code> (i.e., the next lower / higher integer from the base-2 logarithm of ''X'') additional levels to be researched before the next level takes longer to research with the expanded capacity than level <code>M + 1</code> would have taken with the pre-expansion production capacity. | |||
#*For example, if one expands production capacity by a factor of 10, they will be able to research at least <code>floor(log[2](10)) = 3</code> and at most <code>ceiling(log[2](10)) = 4</code> additional levels in a given technology before the exponential increase in price wipes out the speed benefits of their ×10 capacity expansion. | |||
#The cumulative price of the first <code>N - F</code> levels of infinite technologies whose underlying equation is an arithmetic series (equation type (3)) is <code>(N - F) × (P[N] + P[F + 1]) ÷ 2</code>; i.e, <code>N - F</code> times the mean of the prices of the first and last "infinite" level. | |||
#*Expanding production capacity by a factor of ''X'', as above, will in this case allow an additional <code>N × (X - 1)</code> levels to be researched before the benefit of the expansion is wiped out (i.e., research progress speed drops to or below what it was pre-expansion). | |||
#The cumulative price of the first '''''N''''' levels of '''artillery shell shooting speed''', the sole infinite technology whose underlying equation is a powers-of-three geometric series (equation type (2)) is <code>1.5 × P[N] - 0.5 × P[1]</code>; i.e., 1.5 times the price of the final researched level, less half the price of the first level. | |||
#*''Note that the expressions above have been simplified to reflect the fact that this particular technology has F = 0. Since it is the only technology with this equation type, the loss of generality does not matter.'' | |||
:In all calculations above, the constant <code>C</code> (see equations) is ignored; for the technologies where <code>C ≠ 0</code>, results must be adjusted by adding <code>C × (N - F)</code>. This only applies to '''follower robot count''' and '''artillery shooting speed'''. | |||
In all cases, the player can calculate the research price of their target level using the general equations (1), (2), (3) (see preceding sections), look up the price of the first "infinite" level in the table above, then use the summation properties described herein to arrive at a total science pack budget. | |||
Note that these prices reflect '''research units''', which will not be equal to science packs if [[productivity module|productivity modules]] are used in labs. (In that case, the science pack requirement will be lower.) | |||
== All technologies == | == All technologies == | ||
This is a list of all technologies that are currently researchable in the game. | This is a list of all technologies that are currently researchable in the game. | ||
{{TechTable}} | {{TechTable}} | ||
Revision as of 12:47, 3 September 2018
Technologies are researched in labs using science packs.
Every technology needs a certain amount of science packs per science pack type to be researched, for example the Logistics technology requires 20 science pack 1 to be placed in labs while the technology is selected for research.
Achievements

|
Tech maniac Research all technologies. |
- Completing infinite technologies of any level is not required for Tech maniac. All non-infinite levels of technologies that have infinite continuations are still required.
Infinite technologies
While most technologies in Factorio are either one-off or have a finite, relatively small number of levels available, 14 are "infinite", meaning the player can research as many levels as they can afford. All of them unlock bonuses to existing technologies, never new structures or abilities. The per-level bonuses are constant for a particular infinite technologies and, like finite research bonuses, are additive within a single technology.
All infinite technologies levels require space science packs, and are also the only technologies that do. As such, they are late-game technologies intended primarily for players who wish to continue playing and expand their factory past the nominal victory condition of launching a single satellite inside a rocket.
Infinite technologies are identified in-game by a small ∞ infinity symbol shown in the top right corner of the research technology's card in the research screen.
Most infinite technologies are continuations of ordinary multi-level technologies; the "infinite" mechanic becomes effective once the player reaches the card initially labeled with N - ∞ in the research tree. Only the two artillery-related technologies (artillery shell range and shooting speed) are infinite-only; for these, 1 - ∞ is shown before any levels in them are researched. In either case, once the first infinite level is researched, the card label switches to the one discussed above.
Pricing equations
The price of all infinite technologies is generated in a mathematical progression; for the majority of technologies, the progression is geometric, mostly in powers of 2; a single technology - artillery shell shooting speed - uses a powers-of-3 progression. Two technologies - mining productivity and follower robot count - use an arithmetic progression instead, in both cases with a difference of 100.
The equations that generate infinite research prices take the following forms:
P[N] = P[0] × 2 ^ (N - N[0]) + C- most infinite technologiesP[N] = P[0] × 3 ^ (N - N[0]) + C- artillery shell shooting speedP[N] = P[0] × (N - N[0]) + C- mining productivity and follower robot count
where:
- P[N] is the price of the Nth level of the technology (as reported by the game; i.e., counting also all starting "non-infinite" levels, if any)
- P[0] is a price multiplier
- N is the level of the technologies, as reported by the game (i.e., including any starting non-infinite levels)
- N[0] is a shift factor relative to the source series
- C is a constant
Among others, the following properties can be observed:
P[0] = 1,000for technologies with a geometric progression andP[0] = 100for technologies with an arithmetic progression- for both infinite-only technologies,
P[1] = 2 × P[0] - denote
Fthe final level of non-infinite research available in the technology if such exists; for infinite-only research, setF = 0; then: - the majority of geometric-progression technologies have
P[F+1] = P[0]; the rest haveP[F+1] = 2 × P[0](where P[F+1] is the price of the first "infinite" level) - for technologies with preceding non-infinite levels, the relation between P[F+1] and non-infinite level prices may vary, but in all cases
P[F+1] >= P[F](where P[F] is the price of the last non-infinite level) - either
N[0] = ForN[0] = F + 1; this is mostly idiosyncratic to the technology in question C != 0only for follower robot count (C = 900) and artillery shooting speed (C = 1,000)
Price table
The table below summarizes the applicable equation type and parameters (using the same notation as in the preceding section), plus the per-level bonus, for all infinite technologies in the game. Technologies are ordered based on similarity of the parameters; if parameters are identical, then alphabetically.
| Technology | Equation type |
F + 1 | P[F + 1] | Equation | Bonus |
|---|---|---|---|---|---|
| Gun turret damage | 1 | 7 | 1,000 | 1,000 × 2^(N - F - 1) | +70% |
| Rocket damage | 1 | 7 | 1,000 | 1,000 × 2^(N - F - 1) | +50% |
| Bullet damage | 1 | 7 | 1,000 | 1,000 × 2^(N - F - 1) | +40% |
| Shotgun shell damage | 1 | 7 | 1,000 | 1,000 × 2^(N - F - 1) | +40% |
| Flamethrower damage | 1 | 7 | 1,000 | 1,000 × 2^(N - F - 1) | +20% |
| Worker robot speed | 1 | 6 | 1,000 | 1,000 × 2^(N - F - 1) | +65% |
| Combat robot damage | 1 | 6 | 1,000 | 1,000 × 2^(N - F - 1) | +30% |
| Cannon shell damage | 1 | 6 | 1,000 | 1,000 × 2^(N - F - 1) | +30% |
| Laser turret damage | 1 | 8 | 1,000 | 1,000 × 2^(N - F - 1) | +70% |
| Artillery shell range | 1 | 1 | 2,000 | 1,000 × 2^(N - F) | +30% |
| Grenade damage | 1 | 7 | 2,000 | 1,000 × 2^(N - F) | +20% |
| Mining productivity | 3 | 16 | 1,500 | 100 × (N - 1) | +2% |
| Follower robot count | 3 | 7 | 1,000 | 100 × N + 900 | +10 |
| Artillery shell shooting speed | 2 | 1 | 2,000 | 1,000 × 3^(N - F - 1) + 1,000 | +100% |
Affordability
Infinite technologies are essentially an inexhaustible resource sink for players who build very large bases. While the bonuses they provide can significantly improve the player's capabilities (particularly as regards combat), they are subject to diminishing returns; thus, the per-level contributions from very high levels of infinite technologies will eventually provide only marginal improvements.
As the price of most infinite technologies (specifically, those based on geometric progressions) increases very steeply, it may be a good idea for players to set realistic target levels for each of the infinite technologies they wish to pursue, and make their factory plans accordingly. To that end, the following properties of cumulative infinite research prices may be useful:
- The cumulative price of the first
N - Flevels (notation as in previous sections; i.e., here counting "infinite" levels only) of infinite technologies whose underlying equation is a powers-of-two geometric series (see equation type (1) in preceding sections) is2 × P[N] - P[F+1]; i.e., twice the price of the final researched level, less the price of the first "infinite" level.- This tends toward
2 × P[N]as N goes to infinity. - The above also shows that, assuming constant research speed (usually, this is the same as science pack production capacity), each subsequent level of an infinite technology of this type will take about as long as all preceding infinite levels took combined (or, twice as long as the previous level).
- Further, assuming one has reached a level
Mthey consider the "highest feasible" with their current science pack production capacity, expanding said capacity by a factor of X will allow at leastfloor(log[2](X))and at mostceiling(log[2](X))(i.e., the next lower / higher integer from the base-2 logarithm of X) additional levels to be researched before the next level takes longer to research with the expanded capacity than levelM + 1would have taken with the pre-expansion production capacity. - For example, if one expands production capacity by a factor of 10, they will be able to research at least
floor(log[2](10)) = 3and at mostceiling(log[2](10)) = 4additional levels in a given technology before the exponential increase in price wipes out the speed benefits of their ×10 capacity expansion.
- This tends toward
- The cumulative price of the first
N - Flevels of infinite technologies whose underlying equation is an arithmetic series (equation type (3)) is(N - F) × (P[N] + P[F + 1]) ÷ 2; i.e,N - Ftimes the mean of the prices of the first and last "infinite" level.- Expanding production capacity by a factor of X, as above, will in this case allow an additional
N × (X - 1)levels to be researched before the benefit of the expansion is wiped out (i.e., research progress speed drops to or below what it was pre-expansion).
- Expanding production capacity by a factor of X, as above, will in this case allow an additional
- The cumulative price of the first N levels of artillery shell shooting speed, the sole infinite technology whose underlying equation is a powers-of-three geometric series (equation type (2)) is
1.5 × P[N] - 0.5 × P[1]; i.e., 1.5 times the price of the final researched level, less half the price of the first level.- Note that the expressions above have been simplified to reflect the fact that this particular technology has F = 0. Since it is the only technology with this equation type, the loss of generality does not matter.
- In all calculations above, the constant
C(see equations) is ignored; for the technologies whereC ≠ 0, results must be adjusted by addingC × (N - F). This only applies to follower robot count and artillery shooting speed.
In all cases, the player can calculate the research price of their target level using the general equations (1), (2), (3) (see preceding sections), look up the price of the first "infinite" level in the table above, then use the summation properties described herein to arrive at a total science pack budget.
Note that these prices reflect research units, which will not be equal to science packs if productivity modules are used in labs. (In that case, the science pack requirement will be lower.)
All technologies
This is a list of all technologies that are currently researchable in the game.