Tutorial:Applied power math
In this tutorial we'll be answering the question: how much coal is needed to power a factory?
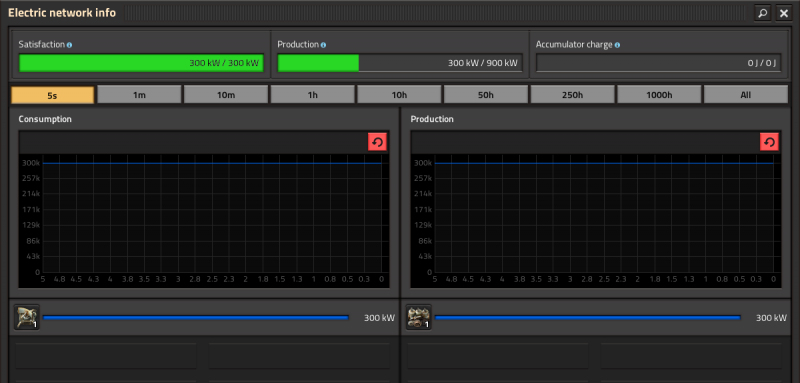
First off, we need to know: how much power does our factory use? That's easy - you can check the electricity tab by clicking on a power pole.
Here we see one radar using 300kW (the 299kW is a rounding error). Factorio uses real science here. A Watt (W) is a measure of energy transfer. A kilowatt (kW) is 1000 W, a megawatt (MW) is 1000 kW, and if you're lucky enough to ever make a factory big enough, a gigawatt (GW) is 1000 MW.
So that answers the question of how much power our factory uses. To keep our factory running at full speed we need to maintain 300 kW. For ease of comparison, we'll convert that to 0.3 MW.
Next question! How much power is stored in coal? That's also easy, because it tells us when we hover over it: 8 MJ.
A Joule is another standard measure of stored energy. As with Watts a kilojoule (kJ) is 1000 J, and so on. There is a fixed relationship between Joules and Watts. 1 Joule can provide 1 Watt of energy for 1 second. As a formula:
J = W × s
So to run our factory at 0.3 MW, we need to consume 0.3 MJ every second.
Quiz
Assuming perfect efficiency, how long could one piece of coal (8 MJ) run our single radar (0.3 MW)?
Expand to reveal answer
8 MJ = 0.3 MW * s
s = 8 MJ / 0.3 MW
s = 26.67
Nearly 27 seconds.
Assuming perfect efficiency, what is the maximum size factory (in watts) a single piece of coal could run for 5 seconds?
Expand to reveal answer
8 MJ = MW * 5
MW = 8 MJ / 5
MW = 1.6
1.6 megawatts.
BONUS! A ton of real world coal about 21 GJ. How much does a piece of Factorio coal weigh?
Expand to reveal answer
The energy ratios will match the weight ratios, so:
8 MJ / 21000 MJ = weight / 1000 kG
weight = (8 MJ / 21000 MJ) * 1000 kG
weight = 0.38 kG
About 400g! Still doesn't explain how our character can carry so much of it...
Efficiency
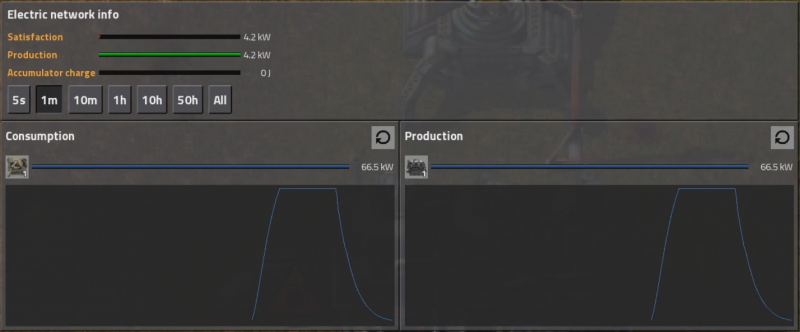
Let's test it! We should be able to run a radar for 27 seconds, right? Using a setup with a single boiler, steam engine, and radar, we can drop a single piece of coal in the boiler and see how long the radar runs.
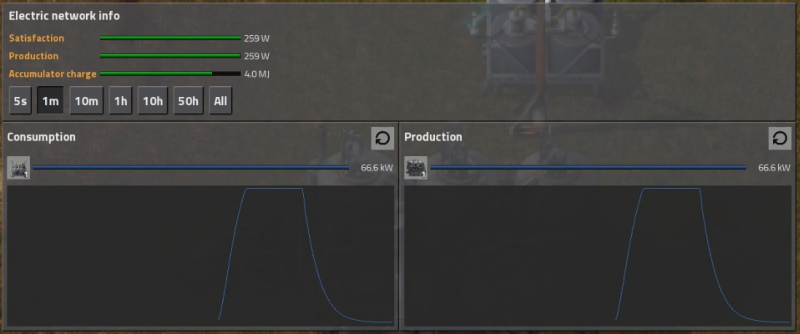
We barely got 20s! What's wrong? We are generating less power than expected. Let's measure using an accumulator.
We started with 8 MJ in the coal, but only 4 MJ ended up in the accumulator. This is because, like in the real world, some energy is lost along the way as waste. In this case, only 50% is making it into our accumulator. In Factorio, this loss happens in the boiler, and it actually tells us this in the item details! You can think of the waste 50% as going into the pollution it generates...
Now we have everything we need to answer our initial question: how much coal do we need to power our factory? Well, the question actually needs to be a bit more precise: how much coal do we need per second to power our factory?
Aside: why did the radar run for 20s and not 13.5s (50% of 27s)?
Because it doesn't turn on and off instantly. As you can see in the graph, it ramps up then ramps down. Technically, if you measured the area under the curve it would give you the expected 4 MJ.
Quiz
How much coal per second is needed to power a 20 MW factory?
Expand to reveal answer
Since J = W × s, a 20 MW factory will consume 20 MJ/s. We'll need 40 MJ/s input due to the 50% boiler efficiency loss. Since coal contains 8 MJ, we'll need 5 coal per second (5 × 8 MJ = 40 MJ).
BONUS! How many mining drills are needed to produce that much coal?
Expand to reveal answer
Per the linked page, an electric mining drill produces ~0.5/s, so we'll need 10 electric mining drills (10 × 0.5 = 5).
SECOND BONUS! The average American household uses GJ in a month (typically expressed as 888 kilowatt hours). How much Factorio coal would be needed to power one of these homes for that period, and how many homes could a steam engine support (510 kW), assuming the power is used at a constant rate? (Assume 30 days in a month.)
Expand to reveal answer
3.2 GJ = 3,200 MJ 3200 MJ / (8 MJ * 50%) = 800
3200 MJ / 30 days / 24 hours / 60 minutes / 60 seconds = 1,235 W 510,000 / 1235 = 412
800 pieces of coal per household, and a single steam engine could power 412 households.